A Test of Aristotle's Eclipse Viewing Method

To test Aristotle's comment that the images of the Sun passing through an aperture are all round I made some geometrically shaped holes in an index card and taped it to the handle of a tripod. The circular hole was made with a hole punch and is 6mm in diameter.


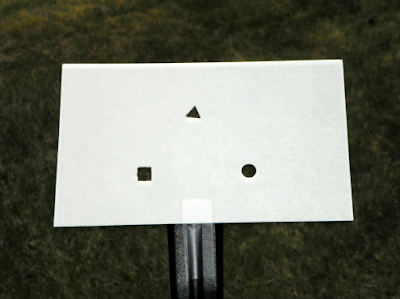
The sunlight passing through the holes was allowed to fall on a white sheet of paper at ground level.


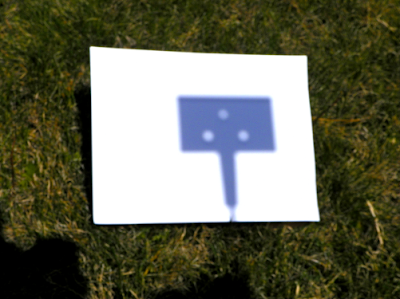
As can be seen all the images are circular. So the shape of the opening is relatively unimportant. It just selects which rays of light are allowed to reach the image. The angular distance of a point on Sun from its center determines the where its image will be located. The blurriness of the image is due to the size of the hole. Note that the image of the triangle is not as bright since its area is slightly smaller.

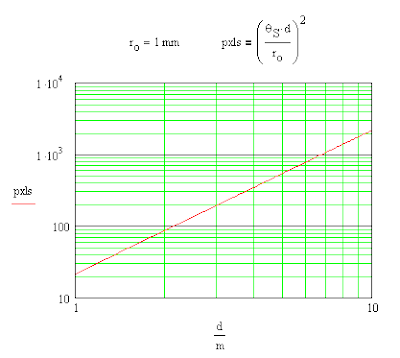
Aristotle's statement that the points that are more distant from the optical axis being less distinct has some truth to it. The brightness of a point in the image depends on how many points of the Sun are cast onto it since the aperture is of finite size. The aperture does a 2D scan of the Sun and its image changes continuously as the position on the screen changes. As one crosses the edge of the Sun fewer points on the Sun contribute to the image brightness as it decreases radially. The edge of the Sun in the image is one "line" wide if a line is defined as the angular size of the aperture relative to the image point.

This is the simpliest method for viewing an eclipse and can be quickly implemented. The size of the aperture can be adjusted to increase or decrease the brightness of the Sun's image. And the size of the image can be magnified by increasing the distance of the screen from the aperture. Since the eclipse in May will last about two hours and end towards sunset the altitude of the Sun will decrease from about 16 degrees to nearly zero degrees and one will have nearly horizontal rays of light to work with. The proximity to the horizon will also filter out some of the sunlight and will ease viewing somewhat. It will make an interesting sunset although it might be a bit of a distraction for some commuters. So if you want to watch the eclipse it might be best to take a "time-out."

Camera Obscura Design (Adding a lens)

For parallel rays passing through a simple lens one finds that the image is produced at the focal distance of the lens and that the angular size of the image is r_d = θ_S f.



In a camera obscura one can use a lens to focus the image and increase the aperture size so that the image is brighter and has more lines of resolution. The drawback is that the focal length of the lens is fixed. Using a 50mm, f = 675mm telescope lens one gets the following results for a camera obscura.


The aperture is relatively large and so one gets a much larger number of lines of resolution for the image. The problem is the small angular diameter of the Sun which makes the image size a little too small. One can try a lens with a longer focal length such as one from a pair of 0.25D computer glasses instead. The image is focused a 4 meters from the aperture and has an angular diameter of 37 mm or about 1.5 inches.


The small width of the computer glasses limits the diameter of the aperture to about 20mm so the number of lines of resolution will be reduced. The problem is finding a suitable low power lens that has a rather long focal length.

Translation of the Magiae Naturalis Passage

Quomodo Solis eclipsis videri possit,
Nunc apponere decreui modum, quo Solis eclipsis clare
notari possit. In Solis eclipsi claude cubiculi fenestras atque
oppones foramini papyrum, et videbis Solem, Speculo
concavoin oppositam papyrum resiliat, et circulum suae
rotunditatis describas, sic initio, medio, et sinefaces. Unde
sene visus laesione diametri puncta Solis defectus notabis.

How the eclipse can be seen,
Now I have decided to set the manner in which an eclipse clearly can be observed. In the eclipse room, shut the windows and opposite the paper opening, and you will see the Sun, the concave mirror bouncing back to the opening in the paper, and his circle defines the round shape, at the beginning, during the middle, and at the end. Thus, while progressing observing a lesion in diametric points of the Sun you will notice the defect.

(Translated with the help of Google Translate. It's probably in need of a little more refinement.)

Readings on the Camera Obscura

 
Camera Obscura - Wikipedia

Camera Obscura - Encyclopedia Britanica, 1902

Aristotle on Eclipses - light from the Sun passing through small openings but similar to Ptolemy's Optics on paraxial rays.

Euclid's Optics - Wikipedia

della Porta - Wikipedia

Magiae Naturalis, Io. Baptistae Portae
Quomodo Solis eclipsis videri possit - Google Translate

Reinhold - Wikipedia

Pinhole Photography - Renner (good book on the subject)

Lord Rayleigh on Pinhole Photography

I just came across an interesting paper by Lord Rayleigh, on Pin-hole Photography, in Philosophical Magazine, Volume 31, 1891. p. 87.

Pinhole Camera Design 3

The diffraction limit depends on the pinhole radius, r_o. It is defined by the angle Δθ = t₀λ/2πr_o where t₀ = 3.832. At the diffraction limit the number of lines equals θ_S/Δθ. Using this result we can solve for r_o in terms of the number of lines which allows us to determine the optimum dimensions for a pinhole camera designed to capture an image of the Sun. For sunlight the wavelength which has the maximum intensity is λ = 555 nanometers.


The results show that the 1 mm pinhole was a little undersized. For 20 lines of resolution the diffraction limit requires r_o = 1.5 mm.

Pinhole Camera Design 2

Here is a recalculation of the design process showing the steps involved a little better.


As you can see there is a trade-off between the number of lines and the illuminance.

Designing a Pinhole Camera

To see if it would be possible to capture a pinhole image of the Sun I decided to try designing a small pinhole camera. One can start out with something with a resolution of 20 lines across which would require about 300 pixels for the image of the Sun. If the pinhole is 1 mm in radius the calculations for the image resolution show that the length of the camera would have to be about 3.5 m long*. This sets the ratio d/r_o = 3500. Checking to see if we have enough light we find that E/E_S = 0.003.* Since the Sun is quite bright we might be able to see this level of illumination under suitable conditions. The diameter of the projected image will be about 3.3 cm.*


To evaluate illumination conditions one would have to check against what one would get by using a pinhole of known size and measuring the illumination at various distances from the pinhole. Photos of the images using a fixed camera setting would help evaluate what a camera and the eye are capable of seeing.

*Edit: I used some data from the old calculations with the 0.1 mm pinhole and noticed that I misread the log scale for the length of the camera. I probably was a little too hasty to post results. Double checking the calculations helps.

Pinhole Images and Their Quality

A pinhole doesn't just pass light through it in proportion the to its area A = πr². There are other effects such as scattering off the sides of the pinhole and diffraction which may be considered proportional to its perimeter P = 2πr, with r being the pinhole radius. The relative flux for the two effects is proportional to the ratio P/A = (2πr)/(πr²) = 2/r which tells us that the perimeter effects dominate for small r. If the pinhole is large enough we can ignore the perimeter effects since the cross-sectional area of a hole increases more rapidly than its perimeter.

For a section of the pinhole image of area dA_i the pinhole acts as an optical stop which blocks some of the light from sections of the illuminating object but allows other sections such as area dA_o to pass through. The light reaching a point on the screen is the sum of the areas passing through and one can apply the laws of photometry to determine the illuminance of a portion of the image.


The quality of the image depends on the relative angular size of the pinhole at the image point and if the distance of the screen, d, is much larger than the radius of the pinhole the angular size is approximately θ = 2r/d. One has to compare this value with the angular size of an actual object in the field of view to determine how detailed its image will be.

Supplemental (Mar 24): I did a plot of the number of pixels for an image of the Sun:


One can see that r_o/d severely limits the image resolution for a pinhole camera.

Scaling Up a Pinhole Camera

Since the angular radius of the Sun is rather small to get a useful image of it one would need a large pinhole camera. One can solve the illuminance formula for the "pinhole" opening which would be needed to keep the same value for the illuminance.


Remember that the image radius is r_d = d·θ_S which makes d = 215·r_d. This suggests a long tubelike pinhole camera for viewing the Sun.

The Pinhole Camera

One can use the flux tube to do radiometric calculations for a pinhole camera in which a small hole in one screen projects light onto a second screen.


At the Earth's surface the sunlight at normal incidence is about 1 kilowatt/meter². We can use this value to calculate the light flux passing through the pinhole. If the pinhole radius is r_o then the flux is calculated as follows.


Since the rays of the Sun diverge by an angle θ_S which is the Sun's angular radius, the approximate radius of the flux tube at distance d is r_d = r_o + d·θ_S.



Knowing the radius of the flux tube we can calculate its area at distance d from the pinhole.


Equating the product E·A = Φ at each end of the flux tube we get the irratiance, E, at distance d.


One sees that as d goes to 0 cm the quantities approach the values at the pinhole. As the distance increases the inverse square law takes over. If one wishes to use a pinhole camera for an eclipse one needs to enclose the image in a box for better contrast and have a side opening of some sort to view the projected image. One can get a sharper image by using a small pinhole but the point of diminishing returns occurs when diffraction takes over. Increasing the distance of the second screen creates a larger but dimmer image.

Numerical Aperture vs F-number

Early this morning I added a correction to the calculation of the concentration of sunlight by a lens in the blog a couple of days ago. The formula could be expressed in terms of the F-number of the lens. A term related to the F-number is that of the Numerical Aperture. Both are connected with the relative angular size formed by an aperture in an optical system. If one thinks of the rim of the Sun and that of its image as stops in the optical system then their angular sizes and "F-numbers" relative to the lens would be the same. Consequently their "numerical apertures" would also be the same. One could possibly simplify the formula for the irradiance of the Sun's image using a "numerical aperture". One would have to think in terms of the angular aperture which would admit the light from the Sun and another that would allow the formation of the image. There seems to be a term missing for this kind of virtual aperture.

Supplemental: There are virtual apertures.

The Plano-Convex Hyperbolic Lens

One can avoid the focusing problems of the biconvex lens associated with its thickness by using a plano-convex lens. Since there is only one refracting surface one needs to double the curvature at the center of the lens so κ₀ = - 2/f where f is the focal length. One can also replace the parameter b by a dimensionless factor β = b/f. This gives the equation for the hyperbolic surface in a more useful form.


The focal point is then determined to an extremely high degree of precision. I encountered problems with the tool used to plot the ray paths due to an insufficient number of data points. The plot below shows the focal point zoomed a billion times and one still cannot observe any deviation from a point focus.


If my value for β is accurate then the dimensions for the focal region are on the order of 10^-15 centimeters which smaller than the diameter of an atom. So the limitations on the precision of the focus seems to depend on how well the lens surface can be shaped and diffraction effects.

I should also point out that I used n = 1 for the index of refraction for the ambient medium and n' = 1.5 for that of the lens. In general the parameter β depends on the two indexes.

Light Concentration by a Lens

We are now in a position to do something that I wanted to do at the beginning of February and that is to determine the light concentrating power of a lens. First we need to redo the plot of the focal region to get a slightly different view. There seems to be some discrepancy in the vertical scale of this plot from the previous one but we will use these values and treat them as nominal.


The rays from the lens come to a focus over a range of 6 μm in the depth of field. The best focus appears to be at just under 5.1 cm from the center of the lens where the radius of the focal area is 15 μm. We can now use the flux tube method to calculate the irradiance of the focal area. If the light passing through the lens has an irradiance of 1 kW/m²* then the flux is the irradiance, E₁, times the transmittance of the lens times its cross-sectional area, A₁. This is equal to the flux passing through the focal cross-sectional area which enables us to determine the irradiance there. This comes to 0.4 gigawatts per square meter which is quite large.


If this energy was absorbed by matter at the focus its temperature would have to be raised considerably so that the radiation emitted would equal the radiation absorbed. We can calculate a concentration factor for the lens, F_C, by dividing the two irradiances. If the focus is too sharp one risks damaging the components of an optical system. This includes the human eye so caution is definitely advised.

These focus computations that involved ray tracing for the spherical, parabolic and hyperbolic lenses used idealized models and Snell's law of refraction. The actual situation is a little more complicated since light is scattered by imperfections in the lens and can be internally reflected at the boundaries of the media. One would expect the sharpness of the focus to deviate somewhat from the idealized model.

*Edit (Mar 21): Deleted the line mentioning the Sun since there was an error for the concentration of light from it. The Sun and its image both have the same angular size but the cross-sectional radius of the image, r_img, is determined by its position which is at the focal length of the lens, f. A corrected calculation for the irradiance of the Sun's image is given below. E_S = 1360 W/m² is the solar constant but the transmission factor T was left at its nominal value of 1.


The result can be expressed in terms of the F-number of the lens. The concentration of sunlight is not as high as was originally indicated but the result is still much larger than the solar constant.

A Hyperbolic Lens

A hyperbolic lens has extremely good focusing qualities. Using the same central curvature and thickness that were used for the spherical and parabolic lenses I was able to get good results by adjusting one free constant. The plots are given in centimeters.


Zooming in on the focal point one can see that the dimensions of the focal region is measurable in micrometers (μm = 10^-6 meters).


The hyperbola's formula, x(y), assumes that the it passes through the origin so one needs to add or subtract a constant to shift it slightly left or right. I was able to eliminate the scale factor, a, for the x axis by solving for a value which gives the curvature, κ₀, at the origin of the function. The remaining scale factor, b, for the y axis was then available to adjust the focusing properties of the lens.

The Aspheric Lens

To avoid the spherical aberration of simple lenses one can use an aspheric lens in which the curvature varies as a function of the radial distance. It can have a positive curvature near the center of the lens and negative curvature near the edges to lengthen the focal length so that the rays all come together at the same point. Another method used with telescopes is the corrector plate.

A Thin Parabolic Lens

The problem that we had with the spherical lens was that the rays furthest from the center did not focus at the same point as the central rays. Instead of a spherical surface one could instead consider a parabolic surface which is not as curved further from the center. A similar calculation for parallel rays passing through a parabolic lens with similar curvature and thickness at its center as we had for the spherical lens shows that the parabolic lens has a better focus. Again the blue dot marks the center of curvature for the left side of the lens and the cyan dot marks the focal point calculated by the lensmaker's equation.


Enlargement of the region about the focus shows that there is still some aberration but the convergence of the rays is better.


Again the difference in position between the center of curvature and the focal point is due to the thickness of the lens. One gets the same converging power for a parabolic lens with the same central curvature. The fact that the outer rays converge too soon tells us that the parabolic lens still has too much curvature as we move away from the center. It would take at least a 4th degree surface to get a better focus than one gets with a parabolic lens.

A Thin Spherical Lens

To understand image formation by a lens one needs to know how the refractive index of glass bends the rays of light along an optical path. Snell's law, n sin(θ) = n' sin(θ'), gives the relation between the angles of the path relative to the surface normal as light goes from one medium to another. Below a set of parallel rays pass from the left through a lens and come to a focus on the right. The lens consists of two identical spherical surfaces with a radius of curvature, ρ = 5 cm, with a thickness, t = 3 mm, which is the maximum distance between the surfaces. The light comes to a focus just beyond the center of curvature to the right marked by the blue dot.


The focal distance, f, computed from the Lensmaker's equation is marked by the cyan dot and the focal point of our thin lens is close to the theoretical value. Looking more closely at the rays coming from the lens one sees that the rays farther from the center converge more closely to the lens than those passing near its center showing what is known as spherical aberration. The majority of the rays come to a focus within 1 mm of the value computed from the formula and the flux of light is greatest there.


The vertical scale is exaggerated somewhat since the lens is a little over 2 cm in diameter and the width of the focal point is actually just a few tenths of a millimeter in diameter. Decreasing the aperature of the lens, the opening about the center through which light is allowed to pass, sharpens the focal point but decreases the amount of light going through it. P = 1/f is known as the converging power of the lens. In this case P = 20.0 diopters (1/#meters) since we were measuring distances in centimeters.*

*edit (Mar 14): Set n = 1.00 which is a better value for air and simplifies the results. Note that in this case the focal length equals the radius of curvature. The difference in position is due to the thickness of the lens.

What Happened to the Aether? 2

In ancient times whether or not the aether exists depended on whether light was considered a wave or to consist of particles. With the Renaisance the question raised itself again. DesCartes and Newton thought of it in terms of particles. Interference phenomena led Huygens develop a wave theory of light. But Maxwell showed that electromagnetism was consistent with the aether being an imcompressible fluid. If the aether was a fliud its density was a constant. This suggested that the aether had a connection with space itself. The Michelson-Morley experiment supported this view.

Wolfgang Pauli stated this in his Theory of Relativity (1958). (Translated from Encyclopdädie der mathematischen Wissenschaften, Vol. V19, 1921.):

"Once the postulate of relativity is stated, the concept of the aether as a substance is thereby removed from the physical theories. For there is no point in discussing a state of rest or of motion relative to the aether when these quantities cannot, in principle, be observed experimentally. Nowadays this is all the less surprising as attempts to derive the elastic properties of matter from electrical forces are beginning to show success. It would therefore be quite inconsistent to try, in turn, to explain electromagnetic phenomena in terms of the elastic properties of some hypothetical medium. Actually, the mechanistic concept of an aether had already come to be superfluous and something of a hindrance when the elastic-solid theory of light was superseded by the electromagnetic theory of light. In this latter the aether subatance had always remained a foreign element. Einstein has recently suggested an extension of the notion of an aether. It should no longer be regarded as a substance but simply as the totality of those physical quanties which are to be associated with matter-free space. In this wider sense there does, of course, exist an aether; only one has to bear in mind that it does not possess any mechanical properties. In other words, the physical quantities of matter-free space have no space coordinates or velocities associated with them."

Quantum Mechanics fudges the question of whether or not light is particle or wave. It is somewhat at odds with Maxwell's electromagnetism. Photons behave like waves with discrete energy and as a result display aspects of both. They are created and annihilated as electrons change state. They can also scatter off electrons. And electrons do not continuously emit radiation as they are accelerated about a nucleus.

One thing that still bothers me about the above is that if one subtracts all the matter from a given region of space is there a remainder? Have we missed something?

What Happened to the Aether?

According to ancient wisdom the world and the heavens were composed of the four elements Earth, Water, Air, Fire and, a fifth element, Aether. Each had its natural place with Earth being the lowest and densest element and the other ranking successively above it with Aether, the lightest and quickest, being above all else*. The Aether was presumed to be what remained when all the other elements were removed in lieu of a Void. Position was achieved through displacement which explained why Fire rose and Earth fell. A substance could have varying proportions of these elements. Aether provided the medium through which light traveled as sound does through the air.

If an aether actually exists its nature must be more complex than that of the air which consists of finely divided particles. Air tends to fill all the space that is available to it. The collisions between particles allows sound to travel from one place to another.

Is the same true for aether and light? There are aether theories that have been proposed to explain the propagation of light. But the analogy of light and sound is not a good one since the properties of light differ from those of sound. Sound which travels through solid matter and air by collisions is a longitudinal wave in which compression and rarefaction take place along the path of travel. Light is a transverse wave and the changes take place normal to the direction of travel. As a result light can be polarized in two directions perpendicular to each other. So if light were carried by some sort of fluid, its aether would have properties different than that of air.

Both electric and magnetic fields can exist in a vacuum so their force fields might be considered properties of a hypothetical aether. But all that we know about electromagnetism are the forces that result from the fields. Maxwell's equations tell us how these fields change in position and time but electromagnetism takes these fields as its foundation in much the same way that Lambert took the intensity of light as his starting point. We don't need to talk about the properties of an aether. Maxwell was able to show that his equations predicted waves which traveled at the speed of light.

The null result of the Michelson-Morley experiment did not immediately do away with the aether. Lorentz was able to reconcile matters with an aether theory by postulating time dilatation and length contraction. But it was gradually realized that reference to an aether was not necessary and the result was Relativity.

For a more thorough discussion of the history involved see:

Maxwell on the Electromagnetic Field, Simpson

A History of the Theories of Aether & Electricity, Whittaker

*Supplemental (Mar 5): How could one justify this hierarchy? Presumably, you have to be quick to stay on top. It's the natural order of things.

Do Flux Tubes Really Exist?

Euler may have been talking about an actual tube in which the flow of fluid took place. Faraday thought that the lines of force had a physical existence. Maxwell thought in terms of an imaginary tube in which a flow existed. What is the actual situation? Are flux tubes are real or imaginary?

We know that an actual barrier has physical properties associated with it. These are the boundary conditions. But what is the situation where no physical boundary exists? There are no boundary conditions acting. But a flow may define a boundary expecially if a "steady" flow exists that doesn't change with time. The sides of the flux tube are defined by the fact that the net flow across the boundary is zero. So in a sense a flux tube exists. It is the boundary that is imaginary.

The component of the forces acting on the fluid normal to an imaginary boundary would have to be zero or there would be an acceleration of the fluid across the boundary. Within a liquid or gas there may be shear forces present but it makes no difference if they are parallel to the surface. The physical barrier of an actual tube wall can also introduce a pressure which counters any forces acting on the fluid. But an imaginary tube has no constraints associated with it.

This is the situation for hydrodynamics dealing with a real fluid. For Electromagnetism and Optics the situation is somewhat less certain. One cannot measure the properties of a hypothetical aether and that makes any aether theory occult. But the dilemma that remains if there is no aether is how do we explain action at a distance for electromagnetic forces? The only rational conclusion is that there is a field present whose nature is unknown. Our knowledge of the nature of the force field is deficient. We can't define a density or velocity of flow for the aether. All that we can observe is an effect on test charges.

For Optics the situation was the same for the flux of light at the time that Photometria was written. Only relative measurements could be made. Today the situation is different and we can define a flux of energy. We can measure the rate at which energy flows across a given surface in a given time. A measurable flux exists for Quantum Optics.

What makes modern Electromagnetism difficult to understand is that a discussion of the aether is suppressed. We have no direct evidence for the existence of an aether. But what we call a "vacuum" may not be a void. It still has physical properties since there are fields present. In Relativity there is frame dragging. In Quantum Mechanics the lowest energy state is not zero energy. In Quantum Optics there is a vacuum state. So the question of the existence of an aether may still be an open one. One can doubt that an aether does not exist. Has the case for its nonexistence been proven?

Euler on Fluid Motion

A paper by Euler, General Principles on the Movement of Fluids (1757), describes the motion of a fluid within a "vessel" which provides the bounds for the flow. He starts by deriving the equations for the flow of an incompressible fluid. In modern vector form the equations are as follows,


There are two equations. The first is a vector equation involving the forces on a element of fluid including the force of acceleration. The second is the scalar continuity equation which gives the condition for the conservation of fluid mass. If the fluid is incompressible, i.e., its density is constant, the the equations of motion can be integrated to give an equation for the direction of flow for the element.

The paper concludes as follows,

"Everything is reduced to finding suitable values ​​for the three velocities u, v, & w, which satisfy our two equations, which contain all that regards our knowledge of the movement of fluids. Since with those three speeds, we can determine the path, that each element of the fluid travels through by its movement. Consider the particle which is at present in Z, and to find the path, it already traveled, and it will travel again, since its three speeds, u, v, & w, are assumed known, we will have to its place in the next moment, dx = u dt, dy = v dt & dz = w dt. We eliminated from these three equalities the time t, and we have two more equations between the three coordinates x, y and z, which seek to determine the path of the fluid element, which is currently in Z, and in general we know the route, that each particle described, and will describe again.

"The determination of these routes is of extreme importance, and must be used to apply the Theory to each case prospect. For if the figure of the vessel, in which the fluid moves, is given, the particles of the fluid, which touch the surface of the vessel, must necessarily follow the direction: & therefore the velocities u, v, & w, must be such that the routes will be deduced to, fall within the same surface. Now we see from what suffices, how we are still far from a complete understanding of the movement of fluids, & that what I have just explained, contains only a weak beginning. But all that which the Theory of fluids includes, is contained in the two equations reported above (§ XXXIV.), so it is not the principles of Mechanics that we are lacking in the pursuit of this research, but only the Analysis, which is not yet adequately cultivated, for this purpose: & therefore it is clear, there are discoveries we have yet to make in this Science, before we can arrive at a more perfect Theory of the movement of fluids."

This paper is sometimes cited as the first reference to tubes of flow. The statement of the problem is not quite the same as that of the imaginary tubes defined by the lines of flow found in Maxwell's works on Electricity and Magnetism and those of hydrodynamics but appears to be more like boundary conditions for the walls of a vessel confining the fluid flow. The boundary conditions usually used today specify that the velocity is zero at the walls of a tube or pipe through which the fluid flows.

This paper by Euler was published only a few years before Lambert's Photometria (1760). Cavendish, who was a Fellow of the Royal Society of London, could be considered a comtemporary of Euler and Lambert although his mathematics does not appear to be as sophisticated.